Cissoid

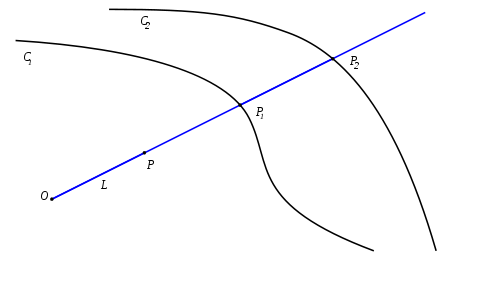
In geometry, a cissoid is a curve generated from two given curves C₁, C₂ and a point O (the pole). Let L be a variable line passing through O and intersecting C₁ at P₁ and C₂ at P₂. Let P be the point on L so that OP = P₁P₂. (There are actually two such points but P is chosen so that P is in the same direction from O as P₂ is from P₁.) Then the locus of such points P is defined to be the cissoid of the curves C₁, C₂ relative to O.

Slightly different but essentially equivalent definitions are used by different authors. For example, P may be defined to be the point so that OP = OP₁ + OP₂. This is equivalent to the other definition if C₁ is replaced by its reflection through O. Or P may be defined as the midpoint of P₁ and P₂; this produces the curve generated by the previous curve scaled by a factor of 1/2.

The word "cissoid" comes from the Greek kissoeidēs "ivy shaped" from kissos "ivy" and -oeidēs "having the likeness of".

Equations

If C₁ and C₂ are given in polar coordinates by r = f₁(θ) and r = f₂(θ) respectively, then the equation r = f₂(θ) − f₁(θ) describes the cissoid of C₁ and C₂ relative to the origin. However, because a point may be represented in multiple ways in polar coordinates, there may be other branches of the cissoid which have a different equation. Specifically, C₁ is also given by

$r=-f_1(\theta+\pi),\ r=-f_1(\theta-\pi),\ r=f_1(\theta+2\pi),\ r=f_1(\theta-2\pi),\ \dots$.

So the cissoid is actually the union of the curves given by the equations

$r=f_2(\theta)-f_1(\theta),\ r=f_2(\theta)+f_1(\theta+\pi),\ r=f_2(\theta)+f_1(\theta-\pi),\$

$r=f_2(\theta)-f_1(\theta+2\pi),\ r=f_2(\theta)-f_1(\theta-2\pi),\ \dots$.

It can be determined on an individual basis depending on the periods of f₁ and f₂, which of these equations can be eliminated due to duplication.

For example, let C₁ and C₂ both be the ellipse

$r=\frac{1}{2-\cos \theta}$.

The first branch of the cissoid is given by

$r=\frac{1}{2-\cos \theta}-\frac{1}{2-\cos \theta}=0$,

which is simply the origin. The ellipse is also given by

$r=\frac{-1}{2+\cos \theta}$,

so a second branch of the cissoid is given by

$r=\frac{1}{2-\cos \theta}+\frac{1}{2+\cos \theta}$

which is an oval shaped curve.

If each C₁ and C₂ are given by the parametric equations

$x = f_1(p),\ y = px$

and

$x = f_2(p),\ y = px$,

then the cissoid relative to the origin is given by

$x = f_2(p)-f_1(p),\ y = px$.

Specific cases

When C₁ is a circle with center O then the cissoid is conchoid of C₂.

When C₁ and C₂ are parallel lines then the cissoid is a third line parallel to the given lines.

Hyperbolas

Let C₁ and C₂ be two non-parallel lines and let O be the origin. Let the polar equations of C₁ and C₂ be

$r=\frac{a_1}{\cos (\theta-\alpha_1)}$

and

$r=\frac{a_2}{\cos (\theta-\alpha_2)}$.

By rotation through angle (α₁ − α2) / 2, we can assume that $\alpha_1 = \alpha,\ \alpha_2 = -\alpha$. Then the cissoid of C₁ and C₂ relative to the origin is given by

$r=\frac{a_2}{\cos (\theta+\alpha)} - \frac{a_1}{\cos (\theta-\alpha)}$

$=\frac{a_2\cos (\theta-\alpha)-a_1\cos (\theta+\alpha)}{\cos (\theta+\alpha)\cos (\theta-\alpha)}$

$=\frac{(a_2\cos\alpha-a_1\cos\alpha)\cos\theta-(a_2\sin\alpha+a_1\sin\alpha)\sin\theta} {\cos^2\alpha\ \cos^2\theta-\sin^2\alpha\ \sin^2\theta}$.

Combining constants gives

$r=\frac{b\cos\theta+c\sin\theta}{\cos^2\theta-m^2\sin^2\theta}$

which in Cartesian coordinates is

x² − m²y² = bx + cy.

This is a hyperbola passing though the origin. So the cissoid of two non-parallel lines is a hyperbola containing the pole. A similar derivation show that, conversely, any hyperbola is the cissoid of two non-parallel lines relative to any point on it.

Cissoids of Zahradnik

A cissoid of Zahradnik (name after Karel Zahradnik) is defined as the cissoid of a conic section and a line relative to any point on the conic. This is a broad family of rational cubic curves containing several well known examples. Specifically:

• The Trisectrix of Maclaurin given by

2x(x² + y²) = a(3x² − y²)

is the cissoid of the circle (x + a)² + y² = a² and the line $x={-{a \over 2}}$ relative to the origin.

• The right strophoid

y²(a + x) = x²(a − x)

is the cissoid of the circle (x + a)² + y² = a² and the line x = − a relative to the origin.

• The cissoid of Diocles

x(x² + y²) + 2ay² = 0

is the cissoid of the circle (x + a)² + y² = a² and the line x = − 2a relative to the origin. This is, in fact, the curve for which the family is named and some authors refer to this as simply as cissoid.

• The cissoid of the circle (x + a)² + y² = a² and the line x = ka, where k is a parameter, is called a Conchoid of de Sluze. (These curves are not actually concoids.) This family includes the previous examples.

• The folium of Descartes

x³ + y³ = 3axy

is the cissoid of the ellipse x² − xy + y² = − a(x + y) and the line x + y = − a relative to the origin. To see this, note that the line can be written

$x=-\frac{a}{1+p},\ y=px$

and the ellipse can written

$x=-\frac{a(1+p)}{1-p+p^2},\ y=px$.

So the cissoid is given by

$x=-\frac{a}{1+p}+\frac{a(1+p)}{1-p+p^2} = \frac{3ap}{1+p^3},\ y=px$

which is a parametric form of the folium.

References

• J. Dennis Lawrence (1972). A catalog of special plane curves. Dover Publications. pp. 53–56. ISBN 0-486-60288-5. 
• C. A. Nelson "Note on rational plane cubics" Bull. Amer. Math. Soc. Volume 32, Number 1 (1926), 71-76.

External links

• Weisstein, Eric W., "Cissoid" from MathWorld.
• 2D Curves
• "Courbe Cissoïdale" at Encyclopédie des Formes Mathématiques Remarquables (in French)
• "Cissoïdales de Zahradnik" at Encyclopédie des Formes Mathématiques Remarquables (in French)