Power of a point

Figure 1. Illustration of the power of point P in the circle centered on the point O. The distance s is shown in orange, the radius r is shown in blue, and the tangent line segment PT is shown in red.

In elementary plane geometry, the power of a point is a real number h that reflects the relative distance of a given point from a given circle. Specifically, the power of a point P with respect to a circle C of radius r is defined (Figure 1)

$h = s^2 - r^2, \,$

where s is the distance from P to the center O of the circle. By this definition, points inside the circle have negative power, points outside have positive power, and points on the circle have zero power. For external points, the power equals the square of the radius of a circle centered on the given point that intersects the given circle orthogonally, i.e., at right angles (Figure 2). The power of a point is also known as the point's circle power or the power of a circle with respect to the point.

The power of a point can be defined equivalently as the product of distances from the point P to the two intersection points of any ray emanating from P. For example, in Figure 1, a ray emanating from P intersects the circle in two points, M and N, whereas a tangent ray intersects the circle in one point T; the horizontal ray from P intersects the circle at A and B, the endpoints of the diameter. Their respective products of distances are equal to each other and to the power of point P in that circle

$\overline{\mathbf{PT}}^2 = \overline{\mathbf{PM}}\times\overline{\mathbf{PN}} = \overline{\mathbf{PA}}\times\overline{\mathbf{PB}} = \left(s - r \right)\times\left(s + r \right) = s^2 - r^2 = h \,$

This equality is sometimes known as the "secant-tangent theorem", "intersecting chords theorem", or the "power-of-a-point theorem".

The power of a point is used in many geometrical definitions and proofs. For example, the radical axis of two given circles is the straight line consisting of points that have equal power to both circles. For each point on this line, there is a unique circle centered on that point that intersects both given circles orthogonally; equivalently, tangents of equal length can be drawn from that point to both given circles. Similarly, the radical center of three circles is the unique point with equal power to all three circles. There exists a unique circle, centered on the radical center, that intersects all three given circles orthogonally, equivalently, tangents drawn from the radical center to all three circles have equal length.

Orthogonal circle

Figure 2: The dashed circle is centered on the point P and intersects the given circle (solid black) at right angles, i.e., orthogonally, at point T. The squared radius of the orthogonal circle equals the power of P with respect to the given circle.

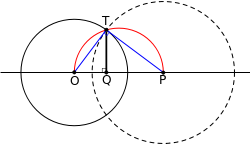
For a point P outside the circle, the power h equals R², the square of the radius R of a new circle centered on P that intersects the given circle at right angles, i.e., orthogonally (Figure 2). If the two circles meet at right angles at a point T, then radii drawn to T from P and from O, the center of the given circle, likewise meet at right angles (blue line segments in Figure 2). Therefore, the radius line segment of each circle is tangent to the other circle. These line segments form a right triangle with the line segment connecting O and P. Therefore, by the Pythagorean theorem,

$R^2 = s^2 - r^2 = p \,$

where s is again the distance from the point P to the center O of the given circle (solid black in Figure 2).

This construction of an orthogonal circle is useful in understanding the radical axis of two circles, and the radical center of three circles. The point T can be constructed—and, thereby, the radius R and the power p found geometrically—by finding the intersection of the given circle with a semicircle (red in Figure 2) centered on the midpoint of O and P and passing through both points. By simple geometry, it can also be shown that the point Q is the inverse of P with respect to the given circle.

Theorems

The power of a point theorem, due to Jakob Steiner, states that for any line through A intersecting C in points P and Q, the power of the point with respect to the circle is given up to a sign by the product

$AP \cdot AQ \,$

of the lengths of the segments from A to P and A to Q, with a positive sign if A is outside the circle and a negative sign otherwise: if A is on the circle, the product is zero. In the limiting case, when the line is tangent to the circle, P = Q, and the result is immediate from the Pythagorean theorem.

In the other two cases, when A is inside the circle, or A is outside the circle, the power of a point theorem has two corollaries.

• The theorem of intersecting chords (or chord-chord power theorem) states that if A is a point in a circle and PQ and RS are chords of the circle intersecting at A, then

$AP \cdot AQ = AR \cdot AS \,$

The common value of these products is the negative of the power of the point A with respect to the circle.

• The theorem of intersecting secants (or secant-secant power theorem) states that if PQ and RS are chords of a circle which intersect at a point A outside the circle, then

$AP \cdot AQ = AR \cdot AS \,$

In this case the common value is the same as the power of A with respect to the circle.

• The tangent-secant theorem is a special case of the theorem of intersecting secants, where points Q and P coincide, i.e.

$AP \cdot AQ = AR \cdot AS \,$

$AP \cdot AP = AR \cdot AS \,$

$AP^2 = AR \cdot AS \,$

This has utility in such applications as determining the distance to a point P on the horizon, by selecting points R and S to form a diameter chord, so that RS is the diameter of the planet, AR is the height above the planet, and AP is the distance to the horizon.

Darboux product

The power of a point is a special case of the Darboux product between two circles, which is given by

$(A_1A_2)^2-r_1^2-r_2^2 \,$

where A₁ and A₂ are the centers of the two circles and r₁ and r₂ are their radii. The power of a point arises in the special case that one of the radii is zero. If the two circles intersect, then their Darboux product is

$r_1 r_2 \cos\varphi \,$

where φ is the angle of intersection.

References

• Coxeter, H. S. M. (1969), Introduction to Geometry (2nd ed.), New York: Wiley .
• Darboux, Gaston (1872), "Sur les relations entre les groupes de points, de cercles et de sphéres dans le plan et dans l’espace", Annales Scientifique de l'École Normale Superieure 1: 323–392 .

• Steiner, Jakob (1826), "Einige geometrische Betrachtungen", Journal für die reine und angewandte Mathematik 1: 161–184 .

Further reading

• Ogilvy CS (1990), Excursions in Geometry, Dover, pp. 6–23, ISBN 0-486-26530-7 
• Coxeter HSM, Greitzer SL (1967), Geometry Revisited, Washington: MAA, pp. 27–31, 159–160, ISBN 978-0883856192 

• Johnson RA (1960), Advanced Euclidean Geometry: An elementary treatise on the geometry of the triangle and the circle (reprint of 1929 edition by Houghton Miflin ed.), New York: Dover Publications, pp. 28–34, ISBN 978-0486462370 

External links

• Jacob Steiner and the Power of a Point at Convergence
• Weisstein, Eric W., "Circle Power" from MathWorld.
• Intersecting Chords Theorem at cut-the-knot
• Intersecting Chords Theorem With interactive animation
• Intersecting Secants Theorem With interactive animation